Model analysis of electroflotation water treatment of wastewater containing microplastics

Ksenofontov Boris Semenovich; Bondarenko Anna Viktorovna; Rusanova Kristina Rodionovna; Ksenofontov Boris Semenovich; Bondarenko Anna Viktorovna; Rusanova Kristina Rodionovna

ISSN: 2455-488X

Journal of Civil Engineering and Environmental Sciences

Mini Review Open Access Peer-Reviewed

Model analysis of electroflotation water treatment of wastewater containing microplastics

Ksenofontov Boris Semenovich*, Bondarenko Anna Viktorovna and Rusanova Kristina Rodionovna

Author and article information

Bauman Moscow State Technical University, 5/1, 2-ya Baumanskaya St., Moscow, 105005, Russia

*Corresponding author: Ksenofontov Boris Semenovich, Bauman Moscow State Technical University, 5/1, 2-ya Baumanskaya St., Moscow, 105005, Russia, Email: ksenofontov@bmstu.ru

doi : 10.17352/2455-488X.000062

Received: 16 May, 2023 | Accepted: 29 May, 2023 | Published: 30 May, 2023

Keywords: Wastewater treatment; Flotation; Process model; Microplastics

Cite this as

Semenovich KB, Viktorovna BA, Rodionovna RK (2023) Model analysis of electroflotation water treatment of wastewater containing microplastics. J Civil Eng Environ Sci 9(1): 014-019. DOI: 10.17352/2455-488X.000062

Copyright License

© 2023 Semenovich KB, et al. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited.

Abstract

The paper presents a model of the microplastic electroflotation process and considers the factors affecting the efficiency of this process during wastewater treatment. The results obtained will help optimize the microplastic electroflotation process and develop more effective ways to remove plastic particles from the treated water, as well as help in the development of new types of flotation technology that allow several purification processes to be carried out simultaneously in one device. These devices can prevent the destruction of the formed flotation complexes, compared with the use of a traditional cleaning scheme with several autonomous devices installed in series. The results obtained provide a rationale for choosing the most efficient electroflotation apparatus for wastewater treatment from microplastics.

Main article text

Introduction

Microplastics (tiny plastic particles less than 5 mm in size) have become a global environmental problem due to their widespread presence in the environment and potentially harmful effects on marine organisms and ecosystems. One of the main sources of microplastics is wastewater, which contains a significant amount of it and enters natural water bodies (rivers, lakes, oceans), disrupting their ecosystems. Thus, the development of effective methods for the removal of microplastics from wastewater is important for the preservation of the health of aquatic ecosystems.

Let’s consider one of the effective ways to remove microplastics from wastewater - flotation. This process involves attaching polluting particles to air bubbles that rise to the surface of the water and can be mechanically removed. However, the effectiveness of microplastic flotation depends on various factors, such as the properties of the microplastic, the chemical composition of the water and the flotation conditions.

In this research paper, we aim to simulate the process of microplastic flotation using electrolysis production of gas bubbles and to investigate the factors affecting its effectiveness. Our results can help optimize the microplastic electroflotation process and develop more effective methods for its removal from wastewater. This is extremely important since the growing use of plastics worldwide has led to the appearance of an alarming amount of microplastics in the environment [1,2]. For example, in the study [3] it is estimated that the oceans alone contain more than 5 trillion microplastic particles. Another study showed that wastewater after treatment facilities can emit up to 4.2 million microplastic particles into the environment per day [4]. The data obtained as a result of the literature review emphasize the need to solve the problem of microplastic pollution of water bodies [5].

Research of a multi-stage model

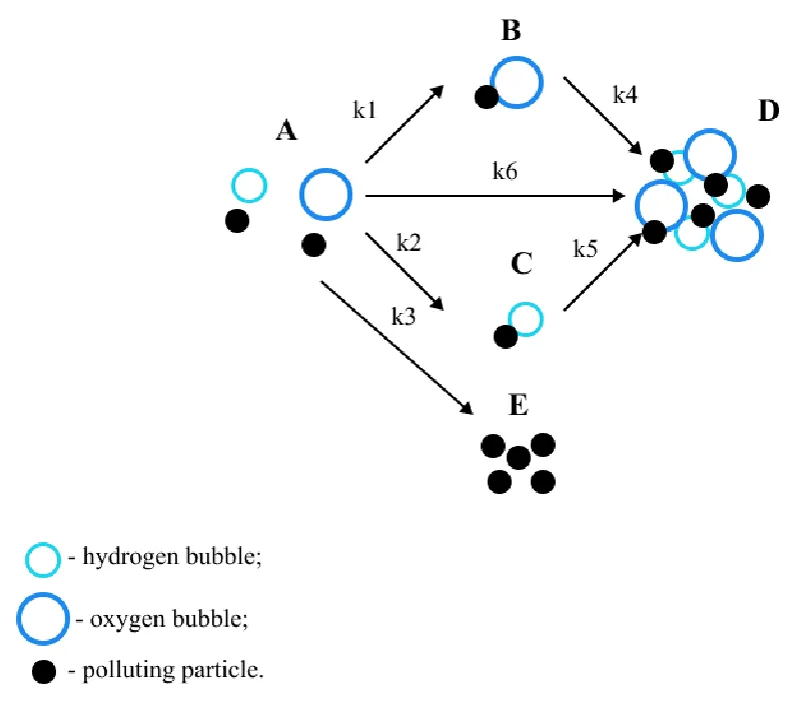
This paper considers B.S. Ksenofontov’s multi-stage model [6-9] of the electroflotation process. The model is presented in Figure 1. Glitter consisting of small plastic particles was chosen as polluting particles, the peculiarities of the material’s behavior in water were taken into account and two different types of bubbles arising during electroflotation were considered [10-12]: hydrogen bubbles and oxygen bubbles [13,14].

In the considered model, state A is the initial state of the system; B and C are the states of adhesion of particles to bubbles; E is the precipitation of particles; D is the state of particles in the foam layer.

The process can be described by a system of differential equations:

${\begin{matrix} \frac{d t}{d} C_{A} = - k_{1} \cdot C_{A} - k_{2} \cdot C_{A} - k_{3} \cdot C_{A} - k_{6} \cdot C_{A}; \\ \frac{d t}{d} C_{B} = k_{1} \cdot C_{A} - k_{4} \cdot C_{B}; \\ \frac{d t}{d} C_{C} = k_{2} \cdot C_{A} - k_{5} \cdot C_{C}; \\ \frac{d t}{d} C_{E} = k_{3} \cdot C_{A}; \\ \frac{d t}{d} C_{D} = k_{4} \cdot C_{B} + k_{5} \cdot C_{C} + k_{6} \cdot C_{A}, \end{matrix} (1)$

Where C_A, C_B, C_C, C_D, C_E – particle concentrations in states А, B, C, D, and E; state А – polluting particles in their original form, В – flotation complexes with oxygen bubbles, С – flotation complexes with hydrogen bubbles, E represents the state of precipitated particles, D is the state of particles in the foam layer; k₁ coefficient describes the probability of formation of the “polluting particle –oxygen bubble” flotation complex, k₂ is the probability of the formation of a “polluting particle – hydrogen bubble” flotation complex, k₃ is the probability of precipitation of a particle from state A; k₄ coefficient characterizes the rising process of the flotation complex “particle–oxygen bubble”, k₅ characterizes the rising process of the flotation complex “particle–hydrogen bubble”, k₆ represents the probability of the particle passing directly into the foam layer.

Initial conditions for this system of equations at t = 0:

$C_{A} (0) = C_{A 0} = 1 m g / l$

$C_{B} (0) = 0$

$C_{C} (0) = 0 (2)$

$C_{D} (0) = 0$

$C_{K} (0) = 0$

The system of equations (1) was solved in the Mathcad software package.

The probability of the formation of a flotation complex is determined by the constants k_1,2, which can be calculated using the formulas [15,16]:

$k_{1} = \frac{1, 5 \cdot k_{э} \cdot j \cdot E}{k_{0} \cdot D_{O_{2}} \cdot ρ_{O_{2}}}; (3)$

$k_{2} = \frac{1, 5 \cdot k_{э} \cdot j \cdot E}{k_{0} \cdot D_{H_{2}} \cdot ρ_{H_{2}}}; (4)$

Where k₃ is the electrochemical equivalent of the substance, kg/C;

j is current density, A/m²;

E is the efficiency of particle capture by a gas bubble during flotation, (DN);

K₀ is bubbles polydispersity factor, (DN);

$D_{H_{2}}, D_{0_{2}}$ , are mean diameters of the bubbles in the flotation cell, m;

ρ is gas density, kg/m³;

q is the bubbling rate, m³/(m² . s).

Sedimentation of microplastic particles is represented in k3 coefficient. To determine the coefficient, an experiment was conducted to observe the deposition of microplastic particles. k3 the coefficient is determined by the formula:

$k_{3} = \frac{v_{s e d}}{H}, (5)$

Where v_sed is sedimentation velocity of a microplastic particle, m/s;

H is the depth of the flotation chamber, m.

Sedimentation velocity is determined by the formula:

$v_{s e d} = \frac{S}{t}, (6)$

Where S is the distance traveled by a microplastic particle, m;

t is the time it took for the particle to travel the distance S, s.

To determine the sedimentation velocity, 10 microplastic particles were observed. Figures 2 and 3 show an example of observation. Table 1 shows the results of observations.

Figures 2 and 3 show an example of a precipitating particle. Table 1 shows the results of observations. According to the results of the experiment, the coefficient k₃ = 0,0036 s^-1 .

The rise of the flotation complexes is characterized by coefficients k_4,5, which can be calculated by the formula:

$k_{4, 5} = \frac{v_{r 4, 5}}{H}, (7)$

Where v_r4,5 is rise velocity of flotation complex, m/s;

H is the depth of the flotation chamber, m.

The transition of the polluting particle into the foam layer is characterized by the k₆ coefficient, which is calculated by the formula:

$k_{6} = \frac{v_{f}}{H}, (8)$

Where vf is the velocity of self-ascent of a microplastic particle, m/s;

H is the depth of the flotation chamber, m.

The determination of the coefficient k₆ according to the formula (8) by the formula (8) is possible if the density of the microplastic is less than the density of water. Since part of the microplastics rose to the surface during the experiment, this assumption was accepted. The particle self-ascent velocity is determined similarly to the sedimentation velocity.

To determine the self-ascent velocity and the coefficient k₆ 10 microplastic particles were observed. Figure 4 shows the initial state of the particle, Figure 5 shows the final state.

The results of the experiment are presented in Table 2. According to the results of the experiment, the value of the coefficient k₆ is 0,0025 s^-1.

The efficiency of particle capture by gas bubbles is determined by the formula [11]:

$E = 0, 5 \cdot \frac{r_{p}^{1, 6}}{r_{b}^{2}} \cdot A^{1 / 6}, (9)$

Where r_ų is particle radius, m;

γ_n is bubble radius, m;

A is the Hamaker constant, J.

In addition to electroflotation, the model takes into account the presence of Al(OH)3 coagulant. The value of the Hamaker constant for this case is given in Table 3.

Table 3: Source data.
Parameter	Value
Hydrogen bubble mean diameter, $D_{H_{2}}$ ,m	52·10^-6
Oxygen bubble mean diameter, $D_{O_{2}}$ ,m	60·10^-6
Microplastic particle diameter, $d_{ч}$ ,m	1·10^-4
Hamaker constant for Al(OH)₃, A, J	12,5·10^-20
Current density, j, mA/cm²	10
The electrochemical equivalent of hydrogen, $k_{H_{2}}$ , kg/C	1,045·10^-8
The electrochemical equivalent of oxygen, $k_{О_{2}}$ ,kg/C	8,29·10^-8
Bubbles polydispersity factor, k₀, (DN)	1
Bubbling rate, q, m³/(m².s)	1,65·10^-5
Depth of the flotation chamber, H, m	1
Hydrogen density, $ρ_{H_{2}}$ , kg/ m³	0,09
Oxygen density, $ρ_{O_{2}}$ , kg/ m³	1,329
Water density, pw, kg/ m³	1000
Water viscosity, m, kg/m·s	1·10^-3

The rise velocity of the flotation complex is determined by the formula [15]:

$v_{r} = \frac{{\bar{D}}^{2} \cdot g \cdot (ρ_{w} - ρ_{g})}{18 \cdot μ}, (10)$

Where $\bar{D}$ is the mean diameter of the bubbles in the flotation cell, m;

ρw is water density, kg/m³;

ρg is gas density, kg/m³;

µ is water viscosity, kg/m·s.

Thus, the presented multi-stage flotation model allows us to consider the influence of various parameters on the efficiency of the process, such as the size of bubbles (depending on the nature of the flotation process), current density, and bubbling rate. In addition, the multi-stage model takes into account various options for the location of pollution particles during the water treatment process.

Calculation of flotation time

For the calculation and graphical representation of the model, a similar process was carried out for the purification of oily wastewater [17,18]. The calculation was made using the initial data presented in Table 3. Reference data is taken from [15,16,18-66].

The parameters calculated by formulas (9), and (10) are presented in Table 4. The obtained values of the constants are presented in Table 5.

Table 4: Calculated process parameters
Parameter	Value
The efficiency of particle capture by hydrogen bubbles, $Е_{H_{2}}$ , (DN)	6,9·10^-2
The efficiency of particle capture by oxygen bubbles, $Е_{O_{2}}$ , (DN.)	5,1·10^-2
Rise velocity of the flotation complex with a hydrogen bubble, $v_{r H_{2}}$ , m/s	1,5·10^-3
Rise velocity of the flotation complex with an oxygen bubble, $v_{r O_{2}}$ , m/s	1,4·10^-3
Sedimentation velocity of microplastic particles, vsed, m/s	0,36·10^-2
The self-ascent velocity of microplastic particles, vf, m/s	0,25·10^-2

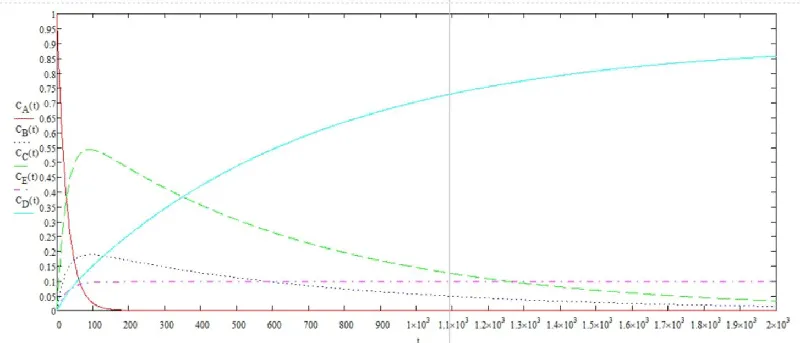
The solution of the system of differential equations in graphical form is shown in Figure 6.

Using the graphical solution, the flotation time was determined. To achieve a degree of purification of 80%, the required flotation time is 1900 seconds.

Conclusion

From the presented flotation process model, it can be concluded that despite the ability of microplastics to independently rise into the foam layer and precipitate other features of the material slow down the flotation time.

Thus, it is necessary to provide additional stages of finer purification, but the use of electroflotation with the ability to adjust the size of bubbles can significantly reduce the concentration of microplastics in water. In the future, the model will be tested experimentally and refined to obtain the final results.

The results obtained in this work provide a rationale for choosing the most efficient electroflotation apparatus for wastewater treatment from microplastics.

References

Article Alerts

Subscribe to our articles alerts and stay tuned.

This work is licensed under a Creative Commons Attribution 4.0 International License.

Quick Enquiry

Table 1:: The results of the particle sedimentation experiment.
Particle number	Time of sedimentation, s	The height of the traversed water layer, m	Sedimentation velocity, m/s	k3, s-1
1	12	0,04	0,0033	0,0033
2	10	0,04	0,0040	0,0040
3	10	0,04	0,0040	0,0040
4	11	0,04	0,0036	0,0036
5	10	0,03	0,0030	0,0030
6	13	0,04	0,0031	0,0031
7	12	0,04	0,0033	0,0033
8	10	0,04	0,0040	0,0040
9	11	0,04	0,0036	0,00363
10	10	0,04	0,0040	0,0040
The average value of the coefficient				0,0036

Table 2: Calculation of the k6 coefficient value.
Particle number	Self-ascent time, s	The height of the traversed water layer, m	Self-ascent velocity, m/s	K6, s-1
1	17	0,04	0,0023	0,0023
2	16	0,04	0,0025	0,0025
3	14	0,04	0,0029	0,0029
4	16	0,04	0,0025	0,0025
5	14	0,04	0,0029	0,0029
6	18	0,04	0,0022	0,0022
7	15	0,04	0,0026	0,0026
8	16	0,04	0,0025	0,0025
9	17	0,04	0,0023	0,0023
10	18	0,04	0,0022	0,0022
The average value of the coefficient				0,0025

Table 5: Calculated coefficients values.
Coefficient	Value
K₁, s^-1	7,93·10^-3
K₂, s^-1	23·10^-3
K₃, s^-1	3,6·10^-3
K₄, s^-1	1,4·10^-3
K₅, s^-1	1,5·10^-3
K₆, s^-1	2,5·10^-3

Journal of Civil Engineering and Environmental Sciences

Model analysis of electroflotation water treatment of wastewater containing microplastics

Ksenofontov Boris Semenovich*, Bondarenko Anna Viktorovna and Rusanova Kristina Rodionovna

Author and article information

Abstract

Indexing and Abstracting

Main article text

Introduction

Research of a multi-stage model

Calculation of flotation time

Conclusion

References

Advertisement

Article Alerts

Table of Contents

Submit your next article Peertechz Publications, also join of our fulfilled creators. Submit a Manuscript

© Peertechz Publications Inc., 10880 Wilshire Blvd., Suite 1101, Los Angeles, California, 90024, USA