Optimization of viscoelastic behaviors of bioinspired asymmetric helicoidal CFRP composites using Taguchi Method

The dynamic mechanical properties play an important role in the selection of suitable materials in the manufacturing wing of aircraft and wind turbine blades. In this paper, the standard Taguchi method was used to examine the effect of inter-ply stacking angles of 0° (UD), 0/90° (cross-ply), 5°, 15°, 10°, 20°, 25° 30° and aging temperature (ambient temperature, 40°, 60°) on the dynamic mechanical properties of bioinspired asymmetric helicoidal Carbon Fiber Reinforced Plastic (CFRP) composites. The standard Taguchi’s L₁₈ was used. The signal-to-noise ratio and analysis of variance were introduced to analyze and estimate the optimal combination parameters. The results show the dynamic mechanical properties are linearly correlated to the fiber architecture and aging temperature. Analysis of variance (ANOVA) indicates that inter-ply stacking angles (15°, and 20°) and aging temperature (40°, 60°) are the main significant factors affecting the dynamic mechanical values at a 95% confidence level. Inter-ply stacking angles are finally noted as critical factors affecting the extent of macromolecular mobility within helicoidally stacked continuous fiber CFRP composites. A confirmation test validated the optimized results and it was found that there were improvements in S/N ratios from initial to optimal setting. The experimental and expected results are very close, with an error ratio not exceeding 5%.

Abbreviations

Coeff: Coefficient; SE Coeff: Standard error Coefficient; T: T-Statistics; P: Probability value; Seq.: Sequential term; Adj. SS: Adjusted Sum of Square; Adj. Ms: Adjusted mean of Square; DF: Degree of Freedom; F: F-Statistics; UD: Unidirectional; H5: 5°; H10: 10°; H15: 15°; H20: 20°; H25: 25°; H30: 30°

Introduction

Composites are materials consisting of at least two materials combined to obtain a material with characteristics different from its constituents, whereas the constituents remain separate and distinct within a composite structure [1]. Helicoidal composite structures in nature are fundamentally asymmetrical and are known to have exceptional tolerance to impact damage [2,3] This architecture consists of stacked laminates of aligned fibers that are rotated by an angle relative to their neighboring laminates to form twisted (Bouligand) architectures with a variety of functions, such as thermal regulation and physical protection [1,4]. In order to reduce fracture, researchers have begun to focus more on the development of helical CFRP as an alternative to typical laminated composite structures [5,6]. The dynamic mechanical performance of helical CFRP, however, has received less attention, even though high-performance composites are commonly used in aerospace and automotive technologies where their viscoelastic and dynamic thermo-mechanical properties are of importance [7,8]. The dynamic mechanical analyzer is an experimental method by which independent anisotropic variables can be examined [9]. While it is known that storage modulus, loss modulus, and glass transition temperature (Tg) vary with inter-ply pitch angle [10-12] there is no report clearly correlating the characteristics these properties as a function of orientation architecture.

For the composite, most of the research focused on experimental tests of the mechanical properties of the composites Muralidhara, et al. [13]; Nwambu, et al. [14] Ekwedigwe, et al. [15]. The correlation between the mechanical properties and the characteristic parameter, e.g., the composition of the composite and the operating conditions is of prime importance for designing proper composites in order to satisfy various functional requirements. Optimization of characteristic parameters on mechanical properties of carbon fiber reinforced composites and finding out the optimal combination of parameters [16]. For the optimization process, among the various techniques such as genetic algorithm, artificial neural network, Taguchi method, and several studies have reported that the Taguchi method has shown a 95% confidence level compared to others [17]. In the Taguchi method, a smaller number of tests is required and it can extract information more precisely and efficiently compared to another approach. Furthermore. it will produce better consistency and reproducibility of results since it uses both a set of standard orthogonal arrays which can be used for many other experimental situations and a standard method for the analysis of results [16]. In this paper, the standard Taguchi method was used to examine the effect of inter-ply stacking angles of 0° (UD), 0/90° (cross-ply), 5°, 15°, 10°, 20°, 25° 30° and aging temperature (ambient temperature, 40o, 60o) on the dynamic mechanical properties of bioinspired asymmetric helicoidal Carbon Fiber Reinforced Plastic (CFRP) composites.

Materials and experimental details

Materials and composite fabrication

MR70 12P carbon fiber prepreg with Toray E750 toughened epoxy resin (Mitsubishi Chemical Carbon Fibre Composites) was vacuum bag manufactured into composite laminates comprising the following different inter-ply orientation angles: 0° (UD), 0/90° (cross-ply), 5°, 15°, 10°, 20°, 25° and 30able 1 provides information on the stacking sequences. While UD and cross-ply composites are symmetric composites, helicoidal composites (50 - 30°) displayed an asymmetrical Bouligand structure. All CFRP composite laminates were manufactured using 20 plies and were 1.9mm thick after curing, which was conducted in a convection oven (Sciquip HT 230), raising the temperature from ambient temperature to 135°C over one hour, holding this temperature for another hour, and then cooling the composite over an hour back to ambient (room) temperature. Table 1 provides information on the stacking sequences used, and the relative fractions of plies oriented between 0° - 45° of the loading axis, and the fraction of those oriented at 46° - 90° of the loading axis [3].

Samples for DMTA testing (3-point bending mode) were prepared and thermal sweep testing was conducted in accordance with ISO 6721-11 [18] using a Triton 2000 Series DMTA. The specimens (n = 3 for each series) were cut to 42 mm (long) and 8.2 mm (wide), a heating rate of 4 °C/min ramped between 50 °C -230 °C at 1Hz at an amplitude of 50 µm. The 3-point bending test span was 15 mm [3].

Experimental design based on the Taguchi method

The experimental procedure is designed with Taguchi’s L₁₈ orthogonal array to reduce the experiment numbers. Taguchi’s L₁₈ orthogonal array contained two columns and eighteen rows, with seventeen degrees of freedom to treat one parameter with three levels and another parameter with six levels. Each machining parameter level is set according to Taguchi’s L₁₈ orthogonal array, based on the Taguchi experimental design method. The experimental observation is further transformed into a signal-to-noise (S/N) ratio using the MINITAB 14 software (Minitab user manual (2003)]. The different levels of variables used in the experiment are given in Table 1.

The S/N ratio for maximum load and stress values can be expressed as the “Higher is better” characteristic, which is calculated as a logarithmic transformation of the loss function. The S/N ratios determined from experimentally observed values are statistically studied by analysis of variance (ANOVA).

Results and discussion

Loss modulus of the CFRP composite

The response surface regression of loss modulus (GPa) versus inter-ply pitch angles and three different aging temperatures (C). The analysis was done using coded units (Table 2).

The investigation looks at the predicted regression coefficients for the loss modulus (GPa) in composites made from carbon fiber-reinforced plastic (CFRP) that are inspired by biological structures. The interactions between the two important variables stacking angles and aging temperature were taken into account. The regression model shows that stacking angles have a substantial negative coefficient of -0.07519 (p = 0.003) influence on the loss modulus of CFRP composites. This shows that changes in stacking orientations can affect how these composites’ dynamic mechanical characteristics behave. This is consistent with earlier research by Muralidhara, et al. [13] that emphasized how important fiber design is in shaping how composite materials behave.

Likewise, the regression model of aging temperature was negative (-0.57000), indicating the impact of aging temperature was statistically significant at the standard significance levels (p = 0.097). The analysis also evaluated interaction effects, revealing that squared stacking angles and aging temperature terms negatively influence the loss modulus. However, like the main effects, these interactions were statistically significant (p > 0.05).

Overall, the model accounts for approximately 66.67% of the variability in the loss modulus, indicating moderate explanatory power [16]. As shown in Table 1, the predicted R-squared value (73.70%) suggests that the model effectively predicts the loss modulus based on the chosen variables.

According to the ANOVA Table 3, the aging temperature and stacking angles have a big impact on the dynamic Loss modulus characteristics of bio-inspired asymmetric helicoidal CFRP composites. The considerable linear effects of both variables (p = 0.003) indicate that variations in stacking angles and aging temperature have a significant effect on the loss modulus [17]. Squared stacking angles, squared aging temperatures, and their interaction, however, show appreciable impact. The low residual error indicates that the model explains a significant percentage of the variability in the loss modulus, despite the fact that the overall model’s significance is only moderately strong (p = 0.187).

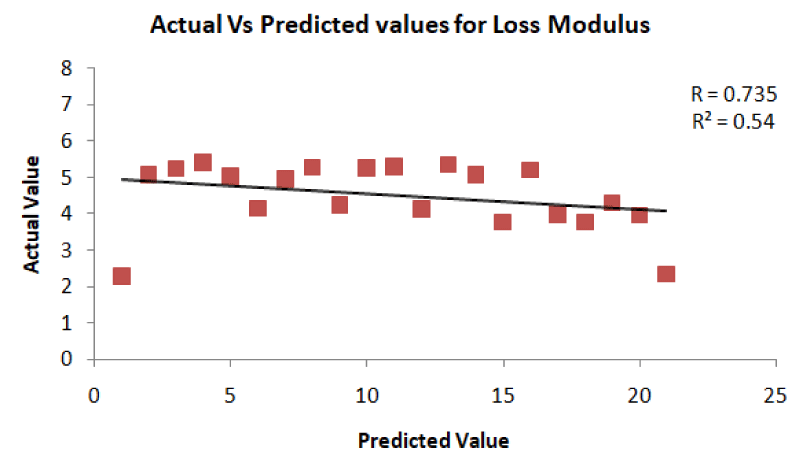
Table 4 presents the forecasted outcomes derived from the regression model, demonstrating that these projections have a 95% likelihood of falling within the lower and upper boundaries, as indicated by the 95% Confidence Interval in Table 3. The model’s predictions resulted in a correlation factor (R) of 0.735 and a coefficient of determination (R2 = 0.54) for the loss modulus (GPa). This suggests that the model’s predictions exhibit an accuracy of approximately 73.5%.

Figure 1, presented that there was a perfect correlation between the actual values and the predicted values, which signifies that there is a very good agreement with the model predictions and experimental results.

Figure 1: Relationship between Actual versus Predicted value for Loss modulus.

Young modulus of CFRP composite

The response surface regression of Young Modulus (GPa) versus Inter-ply pitch angles and aging temp (C).

As presented in Table 5, the estimated regression coefficients for Young Modulus (GPa) in bioinspired asymmetric helicoidal Carbon Fiber-Reinforced Plastic (CFRP) composites show that stacking angles have a minimal influence with a small negative coefficient, but that aging temperature has a significant influence on a significant negative coefficient (p = 0.001). Young Modulus is not considerably impacted by interaction effects, such as squared stacking angles and stacking angles with aging temperature. The model’s high R-squared value (90.3%) and good prediction rate (73.09%) show significant explanatory power. The corrected R-squared value (83.80%) highlights the robustness of the model. According to this, stacking angles are not as important as aging temperature in influencing the stiffness of CFRP composites, which is consistent with Banerjee, et al. [19] and Nwambu et al., [3] studies emphasizing the impact of temperature on CFRP composites.

As presented in Table 6, the analysis of variance (ANOVA) was used to examine the effect of inter-ply stacking angles (0°, 0/90°, 5°, 15°, 10°, 20°, 25°, and 30°) and aging temperature on material properties of bioinspired asymmetric helicoidal Carbon Fiber-Reinforced Plastic (CFRP) composites. With an F-statistic of 3.44 and a p-value of 0.029, the total model was statistically significant, indicating that the combined effects of stacking angles and aging temperature affect the Young Modulus. In particular, the substantial linear effects of stacking angles (p = 0.004) and aging temperature (p = 0.003) showed their influence. Squared terms and interaction effects, however, did not reach statistical significance (p = 0.498). Because the residual error was so small, the model likely accounts for a sizable proportion of the variation in the Young Modulus.

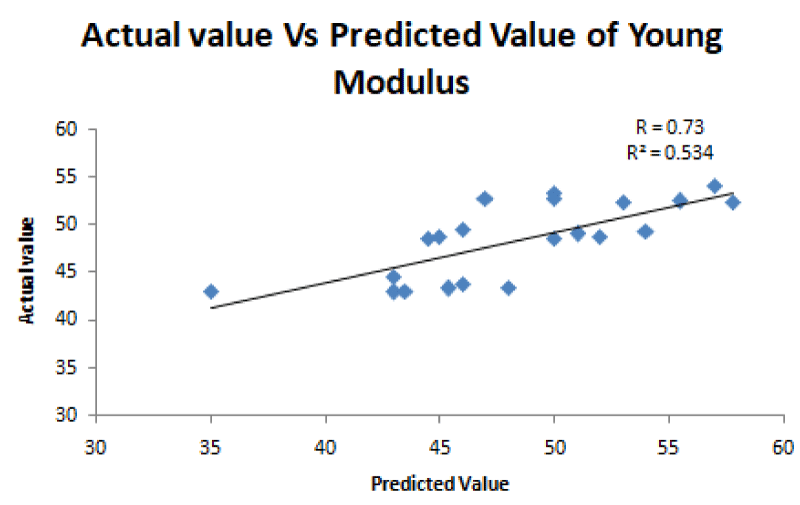
The predicted results from the regression model are shown in Table 7, which also shows that they are 95% likely to fall between the lower and higher boundaries indicated by the 95% Confidence Interval in Table 7. The Young modulus (GPa) was predicted by the model to have a correlation factor (R) of 0.730 and a coefficient of determination (R2 = 0.534). This suggests that the predictions made by the model have an accuracy rate of about 73.0%.

Figure 2 presented that there was a perfect correlation (R) between the actual values and the predicted values, which signifies that there is a very good agreement between the model predictions and experimental results [20,21].

Figure 2: Relationship between Actual versus Predicted value for %Yield.

Conclusion

The following conclusions were obtained: the dynamic mechanical properties are linearly correlated to the fiber architecture and aging temperature. Analysis of variance (ANOVA) indicates that inter-ply stacking angles (15°, and 20°) and aging temperature (40°, 60°) are the main significant factors affecting the dynamic mechanical values at a 95% confidence level. Inter-ply stacking angles are finally noted as critical factors affecting the extent of macromolecular mobility within helicoidally stacked continuous fiber CFRP composites which correspond with the experimental result [3]. A confirmation test validated the optimized results and it was found that there were improvements in S/N ratios from initial to optimal setting. The experimental and expected results are very close, with an error ratio not exceeding 5%.

With the use of the Taguchi optimization method, it was established that fiber architecture and aging temperature are determinant factors in the fabrication of helicoidally stacked continuous fibre CFRP composites though the method could not identify specifically the processing parameters (inter-ply stacking angles) with the highest effect.

1. Alam P. Structures and Composition of the Crab Carapace: An Archetypal Material in Biomimetic Mechanical Design. Results Probl Cell Differ. 2018;65:569-584. doi: 10.1007/978-3-319-92486-1_25. PMID: 30083936.
2. Greenfeld I, Kellersztein I, Wagner HD. Nested helicoids in biological microstructures. Nat Commun. 2020 Jan 13;11(1):224. doi: 10.1038/s41467-019-13978-6. PMID: 31932633; PMCID: PMC6957508.
3. Nwambu CN, Robert C, Alam P. The Tensile Behaviour of Unaged and Hygrothermally Aged Asymmetric Helicoidally Stacked CFRP Composites. Journal of Composites Science. 2022; 6:137.
4. Wen Y, Chen IH, Chen JM, Meyers AM. Flexible Dermal Armor in Nature, The Journal of the Minerals, Metals & Materials Society. 2012; 64:475-485.
5. Ginsburg D, Pinto F, Lervolino O, Meo M. Damage tolerance of bio-inspired helicoidal composites under the low-velocity impact, Composite Structure. 2014;161:187-203.
6. Nwambu C, Robert C, Alam P. Viscoelastic properties of bioinspired asymmetric helicoidal CFRP composites. MRS Advance. 2022. (https://doi.org/10.1557/s43580-022-00332-0)
7. Goertzen W, Kessler M. Dynamic mechanical analysis of carbon/epoxy compositesfor structural pipeline repair, Composites: Part B. 2007; 38:1–9.
8. Zhao C, Zhou Z, Le Guigao. Research on compression properties of unidirectional carbon fiber reinforced epoxy resin composite (UCFREP). Journal of Composite Materials. 2020; 55:11.
9. Melo JD, Radford DW. Time and temperature dependence of the viscoelastic properties of CFRP by dynamic mechanical analysis. Composite Structures. 2005; 70:240-253.
10. Bussu G, Lazzeri A. On the use of dynamic mechanical thermal analysis (DMTA) for measuring glass transition temperature of polymer matrix fibre reinforced composites, Journal of Materials Science. 2006; 41:6072-6076.
11. Coban O, Mustafa OB, Tamer S, and Volkan G. Effect of fibre orientation on viscoelastic properties of polymwer matrix composite subjected to thermal Cycles. Polymer composites. 2010; 411-416.
12. Wegst UG, Bai H, Saiz E, Tomsia AP, Ritchie RO. Bioinspired structural materials. Nat Mater. 2015 Jan;14(1):23-36. doi: 10.1038/nmat4089. Epub 2014 Oct 26. PMID: 25344782.
13. Muralidhara B, Babu KSP, Suresha B. The effect of fiber architecture on the mechanical properties of carbon/epoxy composites. Materials Today: Proceedings. 2020; 22:1755–1764.
14. Nwambu CN, Robert C, Alam P. Dynamic mechanical thermal analysis of unaged and hygrothermally aged discontinuous Bouligand structured CFRP composites. Funct. Compos. Struct. 2022; 4:045001.
15. Ekwedigwe CM, Nwambu CN, Osakwe FO, Nnuka EE. Mechanical Properties of Hybrid Rice Straw Fibre and Walnut Shell ASH Particulate/Epoxy Composite, International Journal of Science and Engineering Invention. 2023: 01-07.
16. Kashan JS. Optimization Using Taguchi Method for Physical and Mechanical Properties of Bio Mimicking Polymeric Matrix Composite for Orthodontic Application,” Engineering and Technology Journal Part A. 2019; 37:5; 181-187.
17. Anmar AO, Hakan K, Farag MM. Effect of reinforcing materials on mechanical properties of composite material using Taguchi method. Journal. Physics: Conference. Series. 2021; 1973 012246.
18. ISO 6721-11:2019. ISO. http://www.iso.org/standard/74988.html
19. Banerjee P, Raj R, Kumar S, Bose S. Tuneable chemistry at the interface and self-healing towards improving structural properties of carbon fiber laminates: a critical review. Nanoscale Adv. 2021 Aug 17;3(20):5745-5776. doi: 10.1039/d1na00294e. PMID: 36132674; PMCID: PMC9419231.
20. Ekwedigwe CM, Nnakwo KC, Nwambu CN. Viscoelastic properties of alkaline treated walnut shell/rice straw fiber/epoxy biocomposite. Journal Civil Engeering and Environmental Science. 2023; 9(1): 009-013.
21. Yang W, Chen IH, Gludovatz B, Zimmermann EA, Ritchie RO, Meyers MA. Natural flexible dermal armor. Adv Mater. 2013 Jan 4;25(1):31-48. doi: 10.1002/adma.201202713. Epub 2012 Nov 15. PMID: 23161399.